Theoretical Analysis of Wind Turbine Tower-Nacelle Axial Vibration Based on the Mechanical Impedance Method

Xiaohui Dong

doi:10.6180/jase.2016.19.1.07

Theoretical Analysis of Wind Turbine Tower-Nacelle Axial Vibration Based on the Mechanical Impedance Method

Mechanical Engineering

Dong Xiaohui This email address is being protected from spambots. You need JavaScript enabled to view it.¹

¹School of Mechanical Engineering, Yancheng Institute of Technology, Yancheng 224051, P.R. China

Received: June 30, 2015
Accepted: November 9, 2015
Publication Date: March 1, 2016

Download Citation: ||https://doi.org/10.6180/jase.2016.19.1.07

ABSTRACT

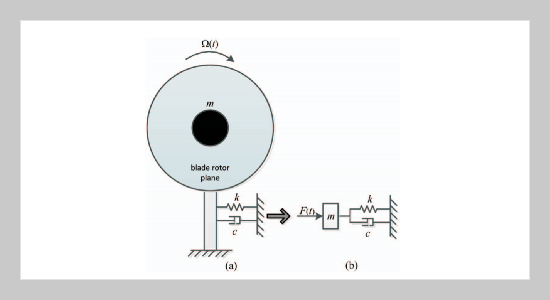
Based on an established axial vibration model of the wind turbine tower-nacelle system, the mechanical impedance method was applied to construct the mechanical network diagram for the axial vibration of the tower-nacelle system. Then, the axial free vibration and forced vibration of the system were analyzed theoretically with considering the displacement impedance or admittance as the transfer function. The analysis shows: for free vibration, the system performs damped vibration with light damping, the amplitude attenuates exponentially with light damping, the system returns to the equilibrium position directly with over-damping, and the system does not generate reciprocating vibration with critical damping; for forced vibration, the amplitude of the axial displacement response is related to the frequency ratio of rotation rate. The resonance frequency does not occur at the undamped natural frequency ω₀. The peak value of the vibration triggered by blade mass imbalance shifts toward the high frequency direction along with the increase of damping ratio ξ, while the peak value of the vibration triggered by tower front spoiler and pneumatic imbalance shifts toward the low frequency direction along with the increase of ξ. If ξ> √2/2 , the amplitude frequency has no peak value, and resonance does not occur. The analysis provides a theoretical basis for the design and control of the wind turbine tower.

Keywords: Wind Turbine, Axial Vibration, Mechanical Impedance Method, Amplitude-frequency Characteristic

REFERENCES

[1] Li, J. F., Cai, F. B., Qiao, L. M., et al., 2014 China Wind Power Review and Outlook, Chinese Renewable Energy Industries Assoication, BeiJing, pp. 110 (2014).
[2] Dai, K. S., Yuan, T. J., Huang, Y. C. and Wang, Y., “A Review of Wind Turbine Tower Structural Safety Studies,” on http//www.paper.edu.cn/releasepaper/ content/201311-37.
[3] De, R. G., Peeters, B. and Ren, W. X., “Benchmark Study on System Identification through Ambient Vibration Measurements,” Proc. of IMAC-XVIII, the 18th International Modal Analysis Conference San Antonio, Texas (2000).
[4] Brincker, R., Zhang, L. and Andersen, P., “Modal Identification from Ambient Responses Using Frequency Domain Decomposition,” Proc. of the 8th International Modal Analysis Conference. Society for Experimental Mechanics, Bethel (2000). doi: 10.1088/0964-1726/10/ 3/303
[5] Foti, D., Diaferio, N. I., Giannoccaro, N. I., et al., “Ambient Vibration Testing, Dynamic Identification and Model Updating of a Historic Tower,” NDT & E International, Vol. 47, pp. 8895 (2012). doi: 10.1016/j. ndteint.2011.11.009
[6] Wang, J. and Hu, X., The Application of MATLAB in Vibration Signal Processing, China Water Power Press, Beijing (2006).
[7] Damgaarda, M., Ibsen, L. B., Andersen, L. V. and Andersen, J. K. F., “Cross-wind Modal Properties of Offshore Wind Turbines Identified by Full Scale Testing,” Journal of Wind Engineering and Industrial Aerodynamics, Vol. 116, pp. 94108 (2013). doi: 10.1016/ j.jweia.2013.03.003
[8] Ma, R. L., Ma, Y. Q., Liu H. Q. and Chen, J. L., “Ambient Vibration Test and Numerical Simulation for Modes of Wind Turbine Towers,” Journal of Vibration and Shock, Vol. 30, No. 5, pp. 152155 (2011) (Chinese).
[9] Velazquez, A. and Swartz, R. A., “Probabilistic Analysis of Mean-response Along-wind Induced Vibrations on Wind Turbine Towers Using Wireless Network Data Sensors,” SPIE Smart Structures and Materials Nondestructive Evaluation and Health Monitoring. International Society for Optics and Photonics, San Diego (2011).
[10] Kusiak, A. and Zhang, Z., “Analysis of Wind Turbine Vibrations Based on SCADA Data,” Journal of Solar Energy Engineering, Vol. 132, No. 3, p. 031008 (2010). doi: 10.1115/1.4001461
[11] Hansen, M. H., Thomsen, K., Fuglsang, P., et al., “Two Methods for Estimating Aeroelastic Damping of Operational Wind Turbine Modes from Experiments,” Wind Energy, Vol. 9, No. 12, pp. 179191 (2006). doi: 10. 1002/we.187
[12] Tcherniak, D., Chauhan, S. and Hansen, M. H., “Applicability Limits of Operational Modal Analysis to Operational Wind Turbines,” Structural Dynamics and Renewable Energy, No. 1, pp. 317328 (2011). doi: 10.1007/978-1-4419-9716-6_29
[13] Ozbek, M. D. R., “Monitoring the Dynamics of Large Wind Turbines in Operation Using Optical Measurement Techniques,” OWEZ IJmuiden, We@Sea (2007).
[14] Zuo, H. S., The Mechanical Impedance Method and Application, China Machine Press, Beijing (1987).
[15] Liao, M. F., Gasch, R. and Twele, J., Wind Power Technology, Northwestern Polytechnical University Press, Xi’an (2009).
[16] Staino, A. and Basu, B., “Dynamics and Control of Vibrations in Wind Turbines with Variable Rotor Speed,” Engineering Structures, Vol. 56, pp. 5867 (2013). doi: 10.1016/j.engstruct.2013.03.014
[17] Robert, G., Klaus, K. and Robert, L., Strukturdynamik, Springer, Verlag Berlin and Heidelberg GmbH & Co. K (2012).
[18] Bianchi, F. D., Battista, H. D. and Mantz, R. J., Wind Turbine Control Systems Principles, Modelling and Gain Scheduling Design, Springer, London (2006).